Free Ramp Calculator Online
In construction and accessibility planning, precision isn’t optional it’s critical. Whether you’re building a wheelchair-accessible entrance, a garden slope, or a loading dock, understanding ramp calculator length, slope angle, and rise-to-run ratios ensures your ramp is functional, safe, and compliant with ADA standards. This is where a ramp calculator, one of the essential construction calculators, becomes indispensable.
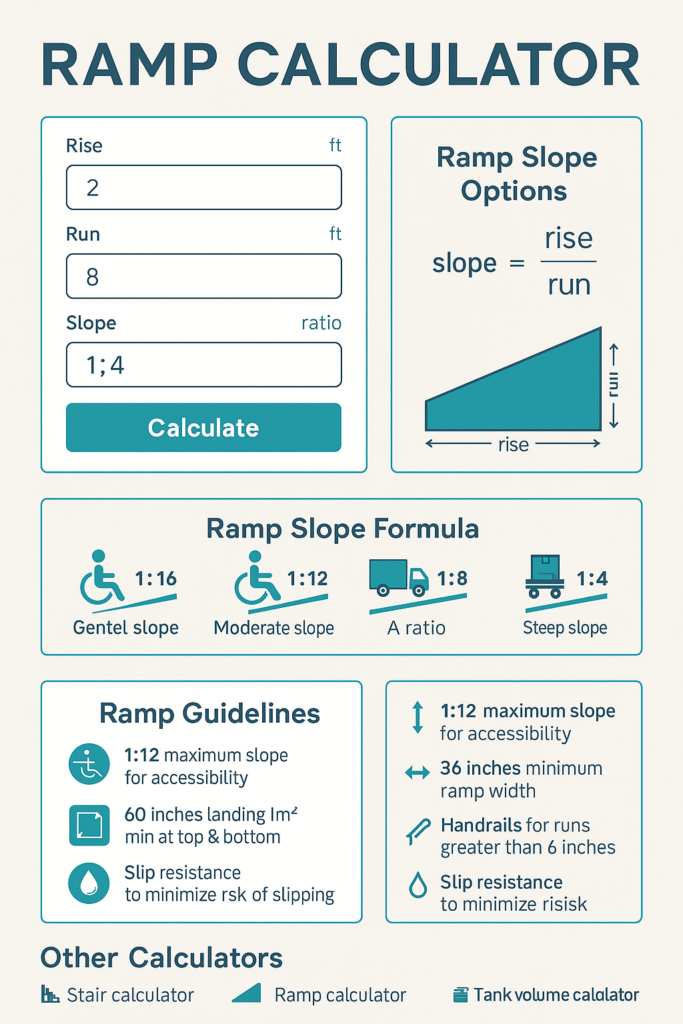
Ramp calculators are digital tools used to calculate the horizontal run, vertical rise, ramp length, and slope angle. With just a few inputs like height, length, and slope you can derive all the necessary parameters. Whether you’re a homeowner installing a ramp for mobility purposes or a contractor adhering to building codes, this tool simplifies your process.
Ramp Slope Calculator
Why Use a Ramp Calculator?
Ramp calculators help ensure ramps meet critical ADA standards, accessibility regulations, and local building codes. These tools eliminate guesswork and convert complex measurements into practical outputs that adhere to industry requirements.
Key benefits include:
- Accurate calculation of ramp length, slope angle, and elevation grade
- Ensures compliance with ADA ramp slope regulations
- Prevents costly errors in ramp construction
- Assists in designing for mobility challenges
- Evaluates purchase price variance based on ramp materials and size
Key Ramp Terminology
Before diving into calculations, it’s helpful to understand common terms used in ramp planning:
- Ramp Length: The total distance from the start of the ramp to the end, along the incline.
- Vertical Rise: The total change in height (in feet or meters) from bottom to top.
- Horizontal Run: The flat distance covered by the ramp’s base.
- Slope Ratio: The incline expressed as a ratio of rise over run, e.g., 1:12.
- Inclination Angle: The degree of steepness.
- Ramp Width: Side-to-side measurement ensuring enough clearance for a wheelchair user.
- Compliance Standards: Rules enforced by organizations like the Americans with Disabilities Act (ADA).
ADA Ramp Standards and Slope Guidelines
The ADA ramp slope standard specifies that for every 1 inch of vertical rise, you must have at least 12 inches of horizontal run, forming a 1:12 slope ratio. This ensures wheelchair ramps are not too steep for accessibility.
ADA-Compliant Ramp Formula:
$$Horizontal Run=12×Vertical Rise\text{Horizontal Run} = 12 \times \text{Vertical Rise}Horizontal Run=12×Vertical Rise$$
For example, if your vertical rise is 30 inches: $$Run=12×30=360 inches=30 feet\text{Run} = 12 \times 30 = 360 \text{ inches} = 30 \text{ feet}Run=12×30=360 inches=30 feet$$
Calculating Ramp Slope, Length, and Angle
A ramp forms a right triangle, where:
- Vertical Rise is one leg,
- Horizontal Run is the other leg,
- Ramp Length is the hypotenuse.
Formula for Ramp Length (using Pythagorean theorem):
$$Ramp Length=(Rise2+Run2)\text{Ramp Length} = \sqrt{(\text{Rise}^2 + \text{Run}^2)}Ramp Length=(Rise2+Run2)$$
Example: $$Ramp Length=(302+3602)=900+129600=130500≈361.2 inches$$ $$\text{Ramp Length} = \sqrt{(30^2 + 360^2)} = \sqrt{900 + 129600} = \sqrt{130500} \approx 361.2 \text{ inches}Ramp Length=(302+3602)=900+129600=130500≈361.2 inches$$
Calculating Ramp Slope Angle
To determine the slope angle, use trigonometric functions.
Ramp Slope Angle Formula:
$$=arctan(Vertical RiseHorizontal Run)\theta = \arctan\left(\frac{\text{Vertical Rise}}{\text{Horizontal Run}}\right)$$ $$θ=arctan(Horizontal RunVertical Rise)$$
Rise-to-Run Ratio
Understanding the rise-to-run ratio helps measure slope steepness.
Rise-to-Run Formula:
$$Slope Ratio=RiseRun\text{Slope Ratio} = \frac{\text{Rise}}{\text{Run}}Slope Ratio=RunRise$$
$$Example: Ratio=30360=112\text{Ratio} = \frac{30}{360} = \frac{1}{12}Ratio=36030=121$$
This 1:12 slope ratio is the standard for most ADA-compliant ramps.
Calculating Horizontal and Vertical Distances
Horizontal Distance:
$$Horizontal Distance=Ramp Length×cos(θ)\text{Horizontal Distance} = \text{Ramp Length} \times \cos(\theta)Horizontal Distance=Ramp Length×cos(θ)$$
Vertical Height:
$$Vertical Height=Ramp Length×sin(θ)\text{Vertical Height} = \text{Ramp Length} \times \sin(\theta)Vertical Height=Ramp Length×sin(θ)$$
These formulas help ensure you’re building ramps with the correct ramp run, vertical rise, and horizontal length.
Example: Full Ramp Design Using All Metrics
Say a building entrance is 24 inches above ground. You want to create a compliant ramp.
Step 1: Calculate Horizontal Run
$$Run=12×24=288 inches\text{Run} = 12 \times 24 = 288 \text{ inches}Run=12×24=288 inches$$
Step 2: Ramp Length
$$Ramp Length=(242+2882)=576+82944=83520≈289 inches\text{Ramp Length} = \sqrt{(24^2 + 288^2)} = \sqrt{576 + 82944} = \sqrt{83520} \approx 289 \text{ inches}Ramp Length=(242+2882)=576+82944=83520≈289 inches$$
Step 3: Slope Angle
$$θ=arctan(24288)≈4.76∘\theta = \arctan\left(\frac{24}{288}\right) \approx 4.76^\circθ=arctan(28824)≈4.76∘$$
These calculations meet ADA standards, ensuring accessibility and compliance.
Wheelchair Ramp Requirements
According to ADA compliance, a wheelchair ramp must:
- Have a maximum slope of 1:12
- Include handrails on both sides if rise exceeds 6 inches
- Provide level landings every 30 feet
- Maintain a minimum width of 36 inches
These parameters directly impact your ramp design, especially in public buildings and residential constructions.
Common Mistakes in Ramp Construction
Even with a solid ramp calculator, errors can occur. Here are frequent pitfalls:
- Misinterpreting ADA slope ratio
- Ignoring local regulations
- Using incorrect units (inches vs feet)
- Overlooking safety clearances
- Failing to account for purchase price variance due to ramp materials
Units & Conversions in Ramp Calculations
To keep calculations consistent, convert all measurements to the same unit inches, feet, or meters.
Inches to Feet:
$$1 foot=12 inches1 \text{ foot} = 12 \text{ inches}1 foot=12 inches$$
Feet to Meters:
$$1 meter=3.28084 feet1 \text{ meter} = 3.28084 \text{ feet}1 meter=3.28084 feet$$
Degrees to Radians:
$$1∘=π180 radians1^\circ = \frac{\pi}{180} \text{ radians}1∘=180π radians$$
Materials & Price Considerations
Material choice affects not just price but also safety and durability. Whether you’re using a construction calculator OSRS to plan your build or a stair calculator to meet accessibility standards, selecting the right materials ensures both cost-efficiency and long-term performance.
- Concrete ramps (durable, non-slip)
- Wooden ramps (affordable, but need maintenance)
- Aluminum ramps (lightweight, easy installation)
When budgeting, consider:
- Length
- Width
- Slope gradient
- Material cost per square foot
This also impacts the purchase price variance, especially in large-scale ramp construction.
Tools and Features of an Online Ramp Calculator
Modern ramp calculators offer more than basic geometry. Features may include:
- Slope angle generator
- Unit conversion tools
- Volume and material estimations
- Accessibility checklists
- Concrete ramp calculator
- Visual renderings and output reports
Integrating Ramp Calculators in Construction Workflows
From residential projects to ADA commercial installations, integrating a ramp slope calculator into your workflow:
- Reduces errors
- Ensures compliance with building codes
- Simplifies bid estimations and material planning
- Streamlines communication between clients, engineers, and contractors
Final Thoughts
A ramp calculator is more than a tool. It is a bridge between design theory and real-world application. Whether you’re working on accessibility standards, ADA ramp slope, or general ramp construction, it ensures your ramp meets the precise requirements set by local and federal laws. As part of the General Construction Calculators, this tool supports safe, compliant, and efficient ramp designs.
Ramp Calculator Reference Table
| Parameter | Formula |
|---|---|
| Ramp Length | $$(Rise2+Run2)\sqrt{(\text{Rise}^2 + \text{Run}^2)}(Rise2+Run2)$$ |
| Horizontal Run | $$12×Vertical Rise12 \times \text{Vertical Rise}12×Vertical Rise$$ |
| Slope Ratio | $$RiseRun\frac{\text{Rise}}{\text{Run}}RunRise$$ |
| Ramp Angle | $$θ=arctan(RiseRun)\theta = \arctan\left(\frac{\text{Rise}}{\text{Run}}\right)θ=arctan(RunRise)$$ |
| Horizontal Distance | $$Length×cos(θ)\text{Length} \times \cos(\theta)Length×cos(θ)$$ |
| Vertical Height | $$Length×sin(θ)\text{Length} \times \sin(\theta)Length×sin(θ)$$ |
| Inches to Feet | $$Inches÷12\text{Inches} \div 12Inches÷12$$ |
| Feet to Meters | $$Feet÷3.28084\text{Feet} \div 3.28084Feet÷3.28084$$ |
