Free Pipe Flow Rate Calculator Online
In any fluid system, from residential plumbing to industrial pipelines, understanding flow rate is critical. Whether you’re analyzing a pipe pressure drop, calculating water velocity, or determining a fluid’s volumetric flow rate, the Pipe Flow Rate Calculator provides engineers, contractors, and students with the right tool to get accurate, fast results.
This guide covers everything from key formulas and cross-sectional area to fluid velocity, pressure loss, and practical examples for different pipe systems and channel shapes.
Pipe Flow Rate Calculator
What Is Flow Rate?
Flow rate refers to the amount of fluid, gas, or liquid that moves through a system per unit of time. It’s typically measured in:
- Cubic feet per second (ft³/s)
- Gallons per minute (GPM)
- Liters per second (L/s)
In engineering and fluid mechanics, flow rate is critical for:
- Designing HVAC systems
- Calculating water supply in circular and trapezoidal channels
- Assessing pressure loss
- Ensuring optimal pipe diameter
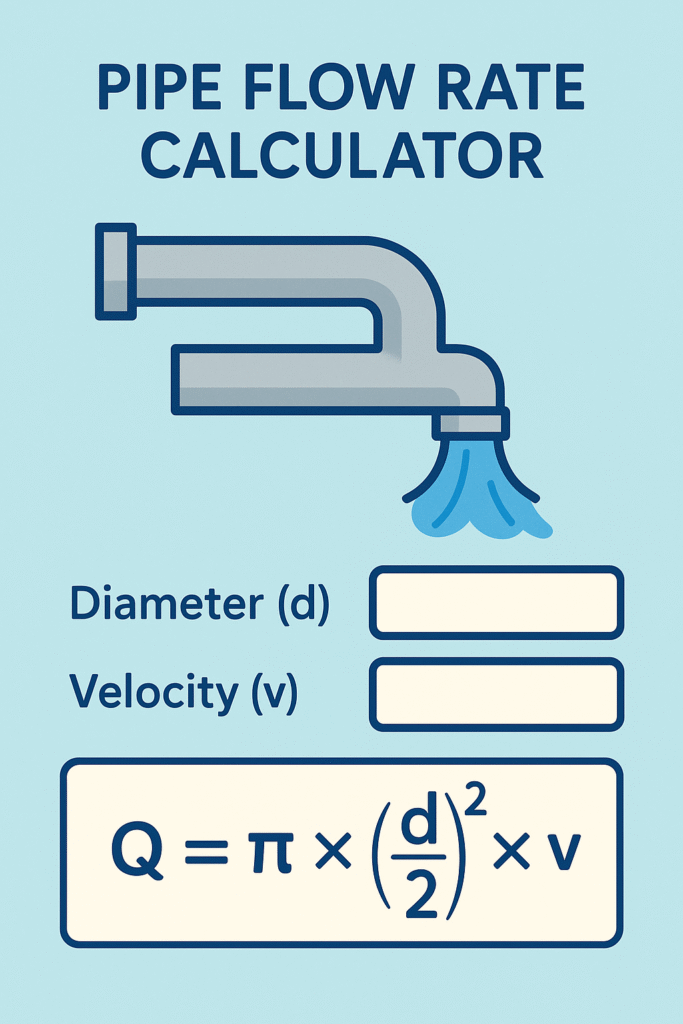
Pipe Flow Rate Formula
To calculate volumetric flow rate: $$Q=A×VQ = A \times VQ=A×V$$
Where:
- QQQ = Volumetric flow rate
- AAA = Cross-sectional area of the pipe or channel
- VVV = Flow velocity
For a full circular pipe: $$A=π×(D2)2A = \pi \times \left( \frac{D}{2} \right)^2A=π×(2D)2$$
Then: $$Q=π×(D2)2×VQ = \pi \times \left( \frac{D}{2} \right)^2 \times VQ=π×(2D)2×V
Use the pipe flow rate calculator to plug in diameter, velocity, and instantly get the flow rate in your desired units.
Example Calculation (Water Through a Pipe)
Imagine water flowing through a circular pipe:
- Pipe diameter: 6 inches = 0.5 feet
- Velocity: 8 ft/s
$$A=π×(0.52)2=0.1963 ft2A = \pi \times \left( \frac{0.5}{2} \right)^2 = 0.1963 \, \text{ft}^2A=π×(20.5)2=0.1963ft2 Q=0.1963×8=1.5704 ft3/sQ = 0.1963 \times 8 = 1.5704 \, \text{ft}^3/\text{s}Q=0.1963×8=1.5704ft3/s$$
Convert to GPM $$(1 ft³ = 7.4805 gallons): 1.5704×7.4805×60=705.2 GPM1.5704 \times 7.4805 \times 60 = 705.2 \, \text{GPM}1.5704×7.4805×60=705.2GPM$$
Your system pushes over 700 gallons per minute. That’s crucial for sizing pumps, valves, and piping.
Important Terms
| Term | Description |
|---|---|
| Flow Rate (Q) | Volume of fluid passing per time unit |
| Velocity (V) | Speed of the fluid (ft/s or m/s) |
| Pipe Diameter (D) | Inner diameter for cross-sectional area |
| Cross-Sectional Area (A) | Surface area the fluid flows through |
| Pressure Drop | Decrease in pressure due to friction or elevation |
| Friction Loss | Resistance due to pipe material and length |
| Density | Fluid’s mass per volume, key in mass flow rate |
| Theta (θ) | Central angle in partially full circular channels |
| Bottom Width | Base of rectangular or trapezoidal channels |
Pipe Flow Rate Calculator Inputs
To use a pipe flow calculator, enter:
- Pipe diameter (inches, mm, feet)
- Flow velocity
- Optional: Friction factor, pressure drop, pipe length
- Select shape: full circular pipe, rectangular channel, or trapezoidal channel
Outputs include:
- Volumetric flow rate
- Mass flow rate
- Pipe pressure drop
- Friction loss
Pipe Flow in Trapezoidal and Rectangular Channels
If your system uses open channels (like ditches or storm drains), cross-sectional shapes vary:
1. Rectangular Channel
A=Width×DepthA = \text{Width} \times \text{Depth}A=Width×Depth
2. Trapezoidal Channel
A=Depth×(Bottom Width+Top Width)/2A = \text{Depth} \times \left(\text{Bottom Width} + \text{Top Width}\right)/2A=Depth×(Bottom Width+Top Width)/2
Use the flow rate calculator with shape selection features to match your actual system and ensure accurate results.
Fluid Velocity Formula
You can reverse-engineer velocity if flow rate and area are known: V=QAV = \frac{Q}{A}V=AQ
For example:
- $$Q = 2 ft³/s$$
- $$A = 0.25 ft²$$
$$V=2/0.25=8 ft/sV = 2 / 0.25 = 8 \, \text{ft/s}V=2/0.25=8ft/s$$
This is helpful in HVAC, hydraulic engineering, and pipeline design.
Calculating Friction Loss
Friction loss reduces pressure and efficiency in any pipe system. Use the Darcy-Weisbach equation: hf=f×LD×V22gh_f = f \times \frac{L}{D} \times \frac{V^2}{2g}hf=f×DL×2gV2
Where:
- hfh_fhf = head loss
- fff = friction factor (depends on Reynolds number)
- LLL = pipe length
- DDD = pipe diameter
- VVV = fluid velocity
- ggg = 9.81 m/s²
Use a friction loss calculator for quick computation.
Pressure Drop and Flow
Pressure drop is influenced by:
- Flow rate
- Pipe diameter
- Pipe length
- Fluid viscosity
- Friction factor
Higher velocity and smaller diameter = $$greater pressure loss. ΔP=f×LD×ρV22\Delta P = f \times \frac{L}{D} \times \frac{\rho V^2}{2}ΔP=f×DL×2ρV2$$
This equation helps in gas systems, HVAC ducts, and high-pressure fluid lines.
Fluid Types and Considerations
Different fluids impact the system differently. Common types include:
- Water: Stable density and low viscosity
- Gases: Compressible; pressure-sensitive
- Oil: Higher viscosity; slower velocity
- Slurries: Abrasive; require special pipe materials
Always specify fluid density, temperature, and system pressure.
Flow Rate in HVAC Systems
In heating and cooling, flow rate helps:
- Size ducts and piping
- Maintain airflow efficiency
- Determine BTU transfer rates
Use the pipe flow rate calculator alongside:
- Reynolds number
- Moody friction factor
- Thermal resistance values
Reynolds Number & Flow Regime
Determine if flow is:
- Laminar (Re < 2000)
- Transitional (2000 < Re < 4000)
- Turbulent (Re > 4000)
Use: $$Re=ρVDμRe = \frac{\rho V D}{\mu}Re=μρVD$$
Where:
- ρ\rhoρ = fluid density
- VVV = velocity
- DDD = diameter
- μ\muμ = dynamic viscosity
This affects your friction loss and pressure drop calculations.
Pipe Flow Rate Use Cases
1. Plumbing
Determine how much water moves through a circular pipe to ensure the system handles demand.
2. Irrigation Design
Know how many GPM are required to supply farms or lawns.
3. HVAC Systems
Calculate airflow or water flow to maintain temperature regulation.
4. Industrial Systems
Size pipes, pumps, and valves for chemical or food production systems.
Conversion Table: Common Units
| From | To | Multiply By |
|---|---|---|
| ft³/s | GPM | 448.831 |
| L/s | m³/h | 3.6 |
| m³/s | L/s | 1000 |
| in | ft | 0.0833 |
| ft² | m² | 0.0929 |
Final Thoughts
The Pipe Flow Rate Calculator is a critical tool in fluid dynamics, engineering design, and system maintenance. It helps calculate:
- Volumetric flow rate
- Fluid velocity
- Mass flow rate
- Friction loss
- Pipe pressure drop
Whether you’re designing a trapezoidal channel, analyzing a full circular pipe, or managing an HVAC loop, this tool gives you the numbers you need to get the job done right.
Summary Table: Key Calculations
| Metric | Formula |
|---|---|
| Flow Rate (Q) | $$Q=A×VQ = A \times VQ=A×V$$ |
| Area (A) | $$π×(D/2)2\pi \times (D/2)^2π×(D/2)2$$ |
| Velocity (V) | $$V=Q/AV = Q / AV=Q/A$$ |
| Head Loss (hf) | $$hf=f⋅(L/D)⋅(V2/2g)hf = f \cdot (L/D) \cdot (V^2 / 2g)hf=f⋅(L/D)⋅(V2/2g)$$ |
| Pressure Drop (ΔP) | $$f⋅(L/D)⋅(ρV2/2)f \cdot (L/D) \cdot (\rho V^2 / 2)f⋅(L/D)⋅(ρV2/2)$$ |
| Reynolds Number (Re) | $$Re=ρVD/μRe = \rho V D / \muRe=ρVD/μ$$ |